Mastering Trajectory: 5 Real-World Applications of Projectile Motion Calculations

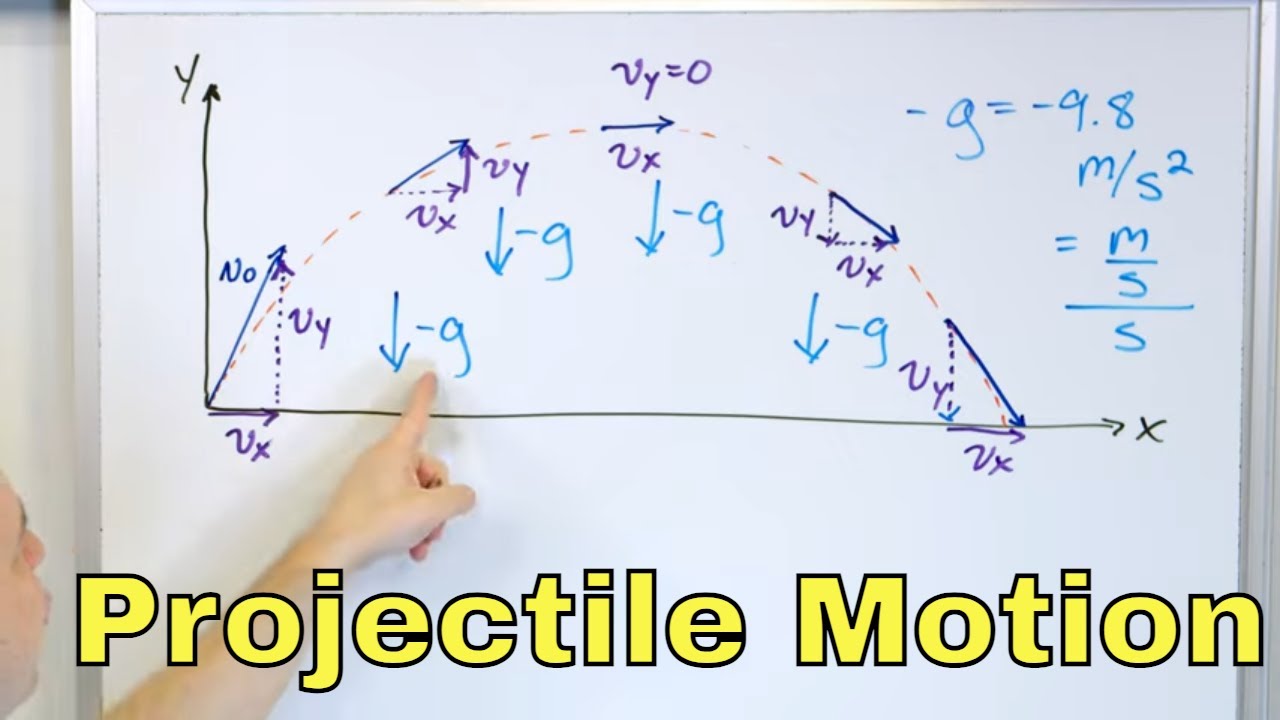
From the graceful arc of a basketball swish to the precise strike of a guided missile, projectile motion governs a surprising number of real-world phenomena. Understanding the principles behind trajectory calculations isn’t just for physics students; it’s a key to unlocking efficiency, precision, and innovation across diverse fields. This article explores five compelling real-world applications where mastering projectile motion makes all the difference.

1. Sports: The Science of the Perfect Throw/Shot

In sports, the trajectory of a projectile – be it a baseball, golf ball, or soccer ball – dictates success. Consider a basketball player attempting a free throw. Factors like launch angle, initial velocity, and air resistance all influence the ball’s path. Coaches and athletes leverage projectile motion calculations, often implicitly, to refine their techniques. A higher launch angle might compensate for a slightly lower initial velocity, increasing the chances of a successful shot. Advanced analytics in sports increasingly rely on precise trajectory modelling to optimize player performance and game strategy.

Sport	Projectile	Key Trajectory Factor
Baseball	Baseball	Spin & Air Resistance
Golf	Golf Ball	Backspin & Launch Angle
Basketball	Basketball	Arc & Release Point

2. Military Applications: Precision Guided Munitions

The military utilizes sophisticated projectile motion calculations for the development and deployment of guided munitions. Factors like wind speed, target movement, and even the Earth’s rotation must be factored into the calculations to ensure accurate targeting. Modern guided missiles use onboard computers and sensors to continuously adjust their trajectory, compensating for unexpected changes in environmental conditions. This ensures pinpoint accuracy, minimizing collateral damage and maximizing effectiveness.

3. Construction and Engineering: Precise Placement of Materials

Construction projects often involve the precise placement of materials, such as concrete or aggregate, across vast distances. Understanding projectile motion is crucial for optimizing the trajectory of materials during processes like pouring concrete from a high-rise building or launching prefabricated components. Careful calculations help minimize waste and ensure the accurate placement of materials, leading to efficiency and safety gains.

4. Fireworks Displays: Choreographing Explosions in the Sky

The breathtaking displays of fireworks rely heavily on meticulously calculated projectile trajectories. Pyrotechnicians must consider factors such as the type of firework, its weight, the powder charge, and wind conditions to determine the optimal launch angle and velocity for each firework. This allows them to create stunning visual effects, ensuring that fireworks explode at precise locations and times to achieve a coordinated and captivating show.

5. Robotics and Automation: Precise Object Manipulation

In robotics and automation, understanding projectile motion is essential for designing robots capable of precisely manipulating objects. Robots need to accurately predict the trajectory of objects they are handling, whether it’s picking up components on an assembly line or placing objects in specific locations. Precise trajectory calculations enable robots to perform tasks with speed and accuracy, improving efficiency and reducing the risk of errors.

Conclusion: From the athletic field to the battlefield and beyond, understanding projectile motion is vital for optimizing performance and achieving accuracy in a multitude of applications. As technology continues to advance, the precision and complexity of trajectory calculations will only increase, further expanding the possibilities for innovation across diverse fields.

Additional Information

Mastering Trajectory: A Deeper Dive into Real-World Applications of Projectile Motion Calculations

The basic principles of projectile motion – incorporating gravity, initial velocity, and launch angle – are deceptively simple. However, their accurate application in real-world scenarios requires a nuanced understanding that goes beyond textbook examples. This analysis delves deeper into five key applications, highlighting complexities and providing additional insights not typically covered in introductory treatments.

1. Ballistics and Weaponry: While calculating the trajectory of a projectile seems straightforward, real-world ballistics are significantly more complex. Factors like air resistance (drag), the Magnus effect (spin-induced lift), and even variations in air density with altitude drastically affect accuracy.

• Air Resistance: Air resistance is not a constant force; it’s proportional to the square of the projectile’s velocity and its cross-sectional area. This nonlinearity necessitates numerical methods like Runge-Kutta integration for accurate trajectory prediction. Ignoring air resistance leads to significant errors, especially at longer ranges and higher velocities. For example, the range of a high-velocity rifle bullet can be underestimated by 50% or more if air resistance is neglected.

• Magnus Effect: The Magnus effect, caused by the spinning of a projectile, generates a force perpendicular to its motion. This is crucial in sports like baseball and golf, where spin is used to control trajectory and increase range or accuracy. For instance, a topspin tennis ball will experience a downward force, causing it to drop more steeply, while a backspin ball experiences an upward force, leading to a higher trajectory. Sophisticated ballistic models incorporate both effects to account for deviation.

• Case Study: The development of precision-guided munitions (PGMs) relies heavily on accurate projectile motion calculations. These systems continuously adjust trajectory based on GPS data, wind speed, and other environmental factors to achieve pinpoint accuracy, compensating for the aforementioned complexities.

2. Sports Science & Performance Enhancement: Optimizing athletic performance relies on understanding projectile motion. Throwing a javelin, kicking a soccer ball, or even the jump shot in basketball all involve projectile motion principles.

• Optimal Launch Angle: The classic textbook angle of 45 degrees for maximum range only applies in a vacuum. Air resistance significantly reduces the optimal angle, making it closer to 35-40 degrees for many real-world scenarios. The optimal angle also varies based on the projectile’s shape and spin.

• Biomechanics: Analyzing the human body’s mechanics in generating projectile motion is critical. Factors such as release velocity, angle, and height, influenced by muscle strength, technique, and body posture, need to be quantified to improve performance. High-speed cameras and motion capture technology are used to collect data for analysis and performance optimization.

• Example: In baseball, analyzing pitcher’s release angle and velocity, combined with batter’s swing speed and bat angle, allows for the prediction of ball trajectory and the development of defensive strategies.

3. Rocketry and Space Exploration: Predicting the trajectory of rockets is essential for successful launches and orbital maneuvers. However, this involves a more complex set of calculations considering the changing mass of the rocket (due to fuel consumption), thrust vectoring, and gravitational variations at different altitudes.

• Tsiolkovsky Rocket Equation: This foundational equation describes the relationship between rocket velocity, propellant mass, and exhaust velocity. Accurate prediction of rocket trajectories requires integrating this equation with other factors like atmospheric drag and gravitational forces.

• Orbital Mechanics: Achieving a stable orbit necessitates precise calculations of gravitational forces and velocity requirements. Small errors in these calculations can result in orbit decay or failure to reach the target orbit.

• Example: The precision required for landing a spacecraft on Mars involves intricate trajectory calculations that account for the gravitational forces of both the Earth and Mars, as well as the effects of solar wind.

4. Civil Engineering and Construction: The trajectory of objects like concrete during pouring or the path of debris during demolition requires careful consideration. This is important for worker safety and structural integrity.

• Concrete Pumping: Understanding the trajectory and velocity of concrete pumped through a long hose is crucial to ensure accurate placement and prevent spills. This involves calculations of pressure, flow rate, and frictional losses within the hose.

• Debris Projection: In demolition projects, it is necessary to predict the trajectory of debris to ensure the safety of workers and surrounding structures. Factors such as explosive force, object weight, and air resistance need to be carefully modeled.

5. Environmental Science and Meteorology: Tracking the trajectory of pollutants in the atmosphere, or predicting the path of a wildfire, uses projectile motion principles, albeit within a complex three-dimensional environment influenced by wind patterns and atmospheric turbulence.

• Air Pollution Dispersion: Understanding how pollutants disperse from a source requires modeling their movement based on wind speed and direction, atmospheric stability, and their settling rates. This helps in regulating emissions and mitigating environmental damage.

• Wildfire Prediction: Predicting the spread of wildfires involves modeling the movement of embers, which behave like projectiles, under the influence of wind. This information is crucial for firefighting efforts and resource allocation.

In conclusion, while the fundamental principles of projectile motion are relatively straightforward, accurately modeling real-world scenarios requires a sophisticated understanding of many contributing factors. Advanced computational methods, coupled with detailed empirical data, are crucial for achieving the level of precision needed across a wide range of applications. Ignoring these complexities can lead to significant errors with potentially serious consequences.