The Mathematical Model Behind Projectile Motion

Unveiling the Secrets of the Sky: A Deep Dive into the Mathematics of Projectile Motion
Projectile motion. The graceful arc of a basketball swish, the terrifying trajectory of a speeding bullet, the elegant parabola of a water fountain’s spray – these are all governed by the same elegant, predictable mathematics. Far from being a dry recitation of formulas, understanding projectile motion unlocks a window into the beautiful interplay of gravity and inertia, a dance as old as time itself.
This article will dissect the mathematical model behind this seemingly simple yet profoundly intricate phenomenon, going beyond the basic equations to reveal the underlying physics and surprising complexities.
The Grand Players: Gravity and Inertia
Before we delve into the equations, let’s meet the stars of our show: gravity and inertia. Inertia, the inherent resistance of an object to changes in its velocity, keeps our projectile moving forward. Gravity, the ever-present force pulling everything towards the Earth’s center, bends its path downwards. The beautiful ballet of projectile motion is simply the result of these two forces acting in concert.
Deconstructing the Trajectory: The Equations
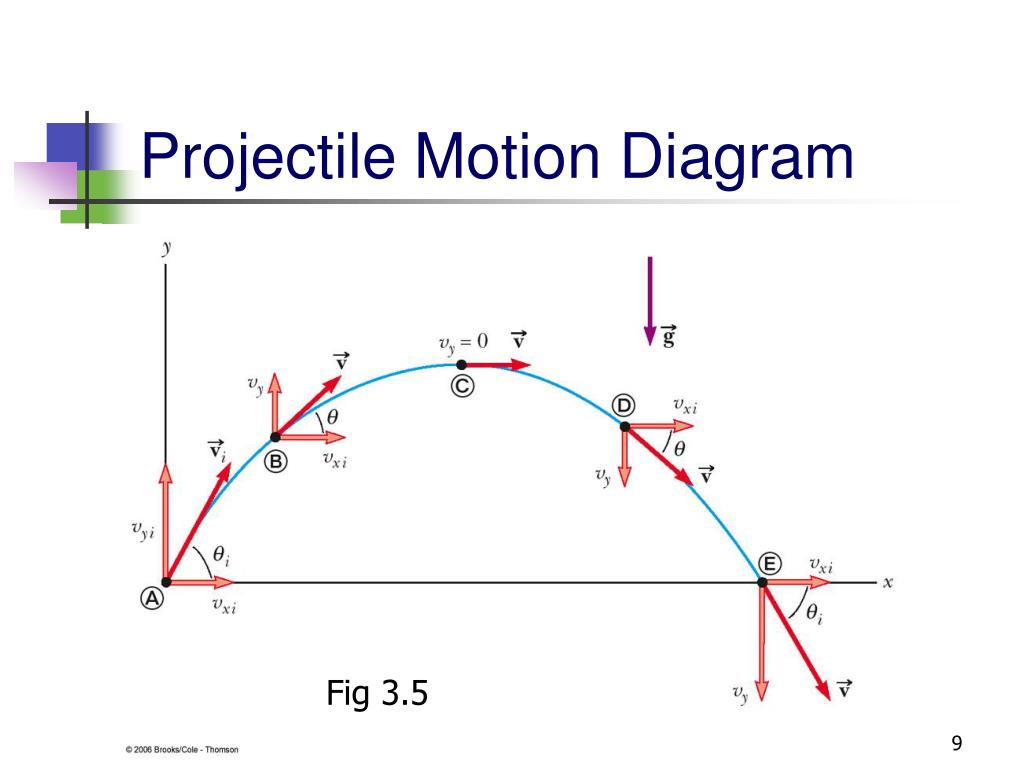
The motion of a projectile can be broken down into two independent components: horizontal and vertical. This simplifies the analysis significantly. Let’s explore the key equations:
Horizontal Motion (assuming negligible air resistance):
- *Distance (x) = v₀ₓ t** where:
- v₀ₓ = initial horizontal velocity
- t = time
This equation tells us that the horizontal velocity remains constant (in the absence of air resistance). The projectile travels the same horizontal distance in each unit of time.
Vertical Motion (affected by gravity):
-
Vertical Velocity (vᵧ) = v₀ᵧ – gt where:
- v₀ᵧ = initial vertical velocity
- g = acceleration due to gravity (approximately 9.8 m/s²)
- t = time
-
Vertical Displacement (y) = v₀ᵧt – (1/2)gt²
These equations paint a vivid picture of the projectile’s vertical journey. Gravity constantly decelerates the upward motion, bringing it to a momentary standstill at the peak of its trajectory before accelerating it downwards.
Beyond the Basics: Factors Affecting Projectile Motion
While the simplified equations provide a solid foundation, real-world projectile motion is significantly more complex. Several factors influence the trajectory:
| Factor | Effect |
|---|---|
| Air Resistance | Slows down the projectile, especially at higher velocities. |
| Wind | Alters both horizontal and vertical velocities, deflecting the trajectory. |
| Spin | Creates aerodynamic forces that can significantly affect the trajectory. |
| Earth’s Rotation | Creates the Coriolis effect, which influences long-range projectiles. |
The Beauty of the Parabola: A Mathematical Marvel
Regardless of the initial velocity and angle, ignoring air resistance, the path of a projectile always follows a parabolic curve. This elegant shape is a direct consequence of the quadratic relationship between vertical displacement and time. The parabola is a testament to the underlying mathematical harmony governing the physical world.
Applications: From Sports to Warfare
Understanding projectile motion isn’t just an academic exercise. It has countless practical applications:
- Sports: From calculating the trajectory of a baseball to optimizing the launch angle of a javelin, projectile motion is crucial for athletic success.
- Military Applications: Accurate artillery fire, missile guidance systems, and the design of weapons all rely heavily on precise calculations of projectile motion.
- Engineering: Designing bridges, launching satellites, and even the flight path of airplanes all involve complex applications of projectile motion principles.
Conclusion: A Continuing Exploration
The mathematical model behind projectile motion is a beautiful and powerful tool. While the simplified equations provide a good starting point, incorporating additional factors like air resistance and wind introduces fascinating complexities that continue to challenge and inspire researchers and engineers alike. The journey into the mathematics of projectile motion is a journey into the heart of physics itself – a testament to the elegant order underlying even the most seemingly chaotic of events.