Unit 2 Vectors Pdf

Unit 2 Vectors Pdf Unit 2 vectors – 2 structure 2.0 introduction 2.1 objectives 2.2 scalar product of vectors 2.3 vector product (or cross product) of two vectors 2.4 triple product of vectors 2.5 answers to check your progress 2.6 summary 2.0 introduction in the previous unit, we discussed vectors and scalars. we learnt how to add and. The vector with initial point p(1,3,2) and terminal point q( 1,0,8) is given by therefore, unit vector in the direction of qp is given by hence, the required vector of magnitude 11 in direction of qp is.

2 04 Vectors Pdf Vectors can be placed anywhere in space. 1 two vectors with the same com ponents are considered equal. vectors can be translated into each other if their com ponents are the same. if a vector ~v starts at the origin o = (0; 0; 0), then ~v = [p; q; r] heads to the point (p; q; r). 2 chapter 2 vectors ito understand the concept of a vector and to apply the basic operations on vectors. ito recognise when two vectors are parallel. ito understand linear dependence and linear independence. ito use the unit vectors i and j to represent vectors in two dimensions. ito use the unit vectors i, j and k to represent vectors in three. Unit vectors a unit vector is a dimensionless vector having a magnitude of exactly 1, and used to specify a given direction and have no other physical significance. we shall use the symbols i, j, and k to represent unit vectors pointing in the positive x, y, and z directions, respectively. Physics grade 11 unit 2 vectors free download as word doc (.doc .docx), pdf file (.pdf), text file (.txt) or read online for free. this document outlines a physics grade 11 unit on vectors that contains 23 questions.

Mm 2021i Class 2 Vectors Pdf Euclidean Vector Trigonometric Unit vectors a unit vector is a dimensionless vector having a magnitude of exactly 1, and used to specify a given direction and have no other physical significance. we shall use the symbols i, j, and k to represent unit vectors pointing in the positive x, y, and z directions, respectively. Physics grade 11 unit 2 vectors free download as word doc (.doc .docx), pdf file (.pdf), text file (.txt) or read online for free. this document outlines a physics grade 11 unit on vectors that contains 23 questions. Quantity with a magnitude and a direction. it can be represented by a vector. examples: displacement, velocity, acceleration. displacement does not describe the object’s path. quantity with magnitude, no direction. ii. on an axis. unit vector: vector with magnitude 1. no dimensions, no units. analytical method: adding vectors by components. In this unit we describe how to write down vectors, how to add and subtract them, and how to use them in geometry. in order to master the techniques explained here it is vital that you undertake plenty of practice exercises so that they become second nature. use vectors to solve simple problems in geometry. 4. some notation for vectors 3. 5. Vectors can be related to the basic coordinate systems which we use by the introduction of what we call "unit vectors." a unit vector is one, which has a magnitude of 1 and is often indicated by putting a hat (or circumflex) on top of the vector symbol, for example . the quantity is read as "a hat" or "a unit". In class i will write these vectors with arrows above them to indicate they are unit vectors, i.e. . ijk,, the component vectors can now be written in unit vector notations as: ax = axi and ay = ayj where the terms ax and ay are the scalar components of the vector a, respectively. recall ax = acos2 and ay = asin2. the following figures show how.

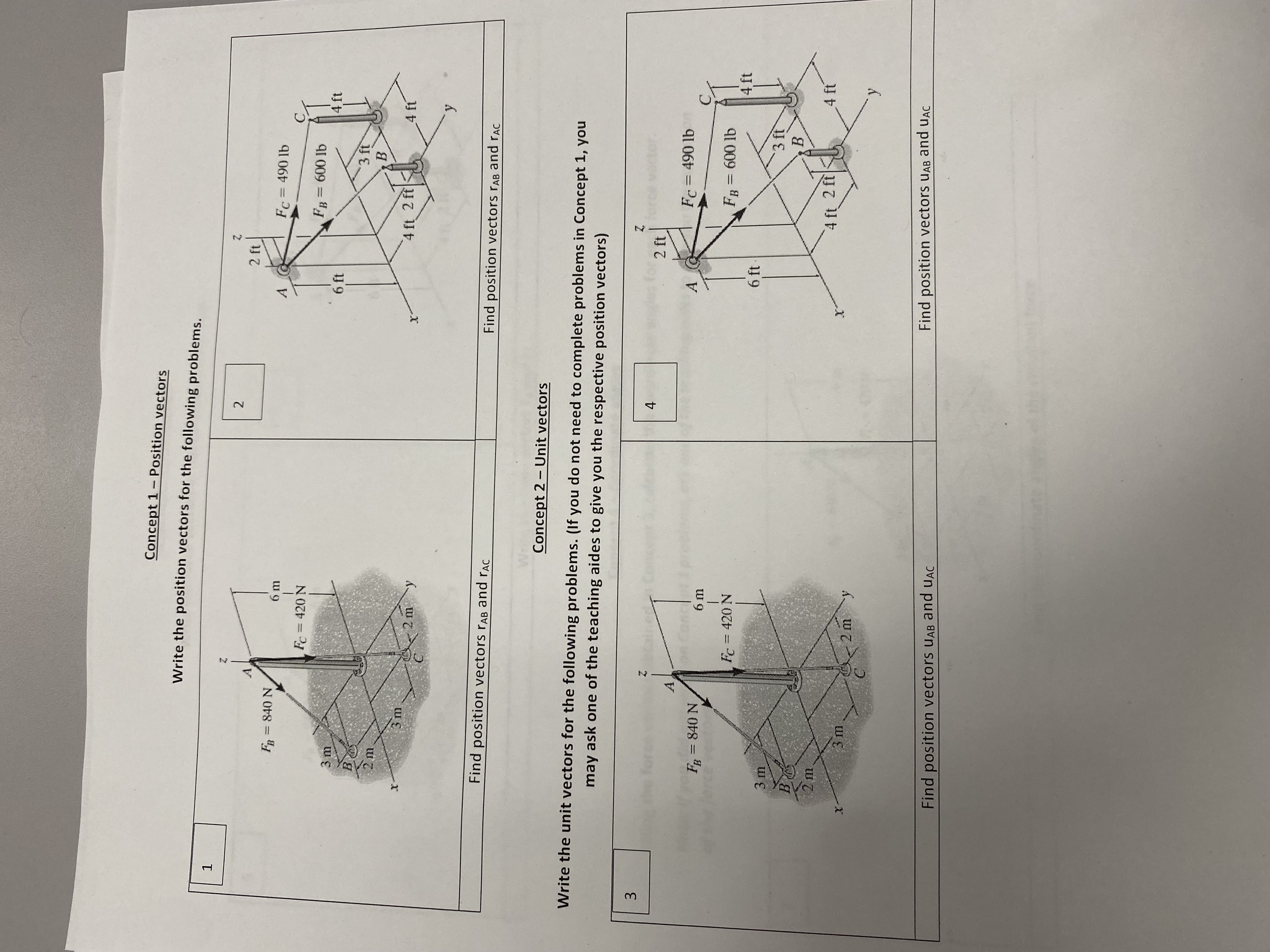
Solved Concept 2 Unit Vectors Write The Unit Vectors For The Chegg Quantity with a magnitude and a direction. it can be represented by a vector. examples: displacement, velocity, acceleration. displacement does not describe the object’s path. quantity with magnitude, no direction. ii. on an axis. unit vector: vector with magnitude 1. no dimensions, no units. analytical method: adding vectors by components. In this unit we describe how to write down vectors, how to add and subtract them, and how to use them in geometry. in order to master the techniques explained here it is vital that you undertake plenty of practice exercises so that they become second nature. use vectors to solve simple problems in geometry. 4. some notation for vectors 3. 5. Vectors can be related to the basic coordinate systems which we use by the introduction of what we call "unit vectors." a unit vector is one, which has a magnitude of 1 and is often indicated by putting a hat (or circumflex) on top of the vector symbol, for example . the quantity is read as "a hat" or "a unit". In class i will write these vectors with arrows above them to indicate they are unit vectors, i.e. . ijk,, the component vectors can now be written in unit vector notations as: ax = axi and ay = ayj where the terms ax and ay are the scalar components of the vector a, respectively. recall ax = acos2 and ay = asin2. the following figures show how.

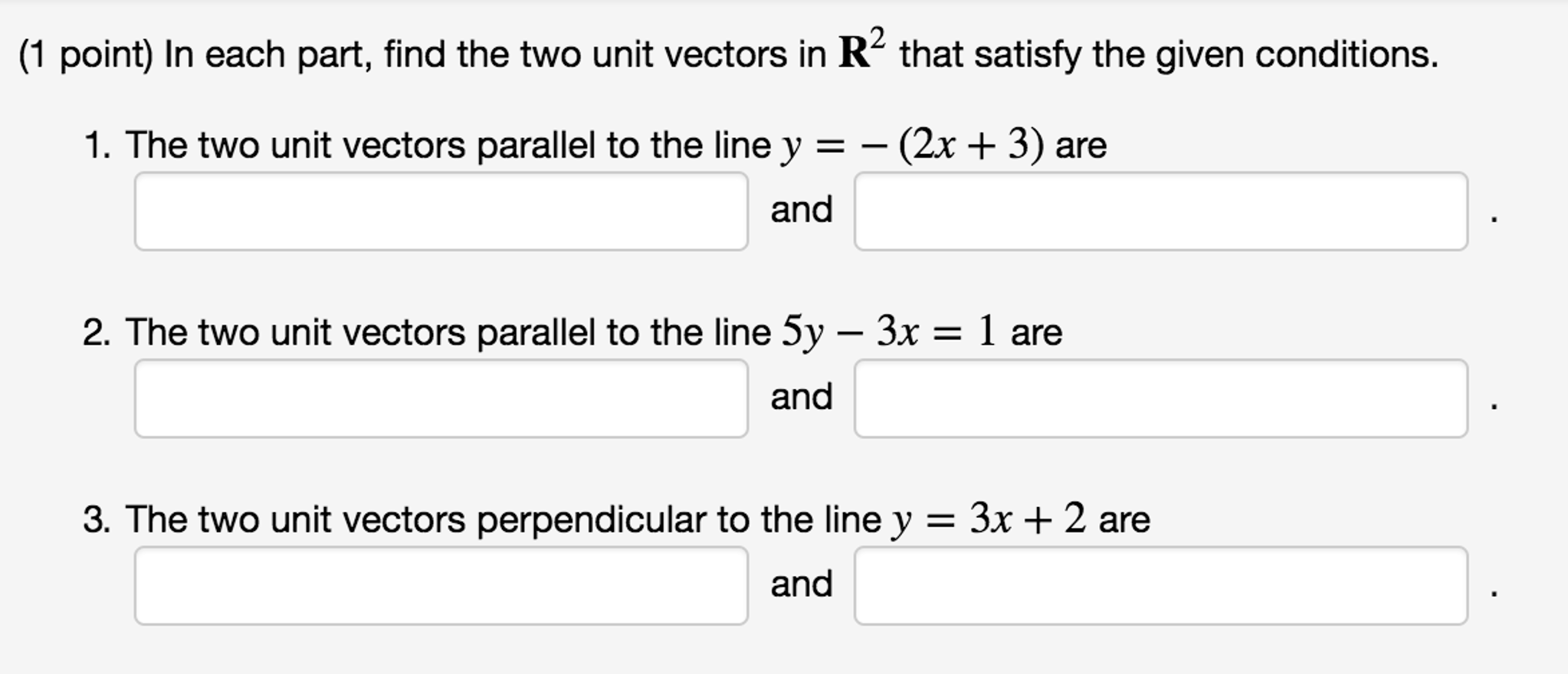
Solved In Each Part Find The Two Unit Vectors In R 2 That Chegg Vectors can be related to the basic coordinate systems which we use by the introduction of what we call "unit vectors." a unit vector is one, which has a magnitude of 1 and is often indicated by putting a hat (or circumflex) on top of the vector symbol, for example . the quantity is read as "a hat" or "a unit". In class i will write these vectors with arrows above them to indicate they are unit vectors, i.e. . ijk,, the component vectors can now be written in unit vector notations as: ax = axi and ay = ayj where the terms ax and ay are the scalar components of the vector a, respectively. recall ax = acos2 and ay = asin2. the following figures show how.

2 Vectors And Unit Vectors Ma 242 Studocu