Vectors Review

Vectors Review Pdf If it has been a while since your last physics lecture, you may need to be refreshed about these concepts. in this section, we will be discussing the basics about vectors and the correlation between position, velocity, and acceleration vectors. Chapter 7.3 the dot product of two geometric vectors. chapter 7.4 the dot product of two algebraic vectors. chapter 7.5 scalar and vector and projections. chapter 7.6 the cross product of two vectors. chapter 7.7 applications of the dot product and cross product. chapter 8. chapter 8.1 vectors and parametric equations of a line in r2.

Free Review Vector Images Over 640 A one page document that covers the basics of vectors, vector operations, trigonometry, and calculus. includes definitions, formulas, examples, and diagrams for easy reference and review. In this (very brief) chapter we will take a look at the basics of vectors. included are common notation for vectors, arithmetic of vectors, dot product of vectors (and applications) and cross product of vectors (and applications). Vectors are added by a head to tail method and the resultant is drawn from the tail of the first vector to the head of the last vector. so if two vectors are added say b is added to a (as in a b) then first a is drawn and the tail of b is placed at the head of a. Review: vectors. review the following videos before starting the course. 0.1 vectors vs. scalars; 0.2 vector operators; 0.3 coordinate systems and unit vectors; 0.4 vectors magnitude and direction; 0.5 vector decomposition into components; 0.6 going between representations « previous | next ».

Review Vector Analysis Pdf Euclidean Vector Cartesian Coordinate Vectors are added by a head to tail method and the resultant is drawn from the tail of the first vector to the head of the last vector. so if two vectors are added say b is added to a (as in a b) then first a is drawn and the tail of b is placed at the head of a. Review: vectors. review the following videos before starting the course. 0.1 vectors vs. scalars; 0.2 vector operators; 0.3 coordinate systems and unit vectors; 0.4 vectors magnitude and direction; 0.5 vector decomposition into components; 0.6 going between representations « previous | next ». In basic engineering courses, the term vector is used often to imply a physical vector that has “magnitude and direction and satisfies the parallelogram law of addition.” in mathematics, vectors are more abstract objects than physical vectors. like physical vectors, tensors must satisfy the rules of tensor addition and scalar multiplication. Review of vectors we will work with real vectors, meaning vectors for which each component is a real number. with minimal changes, one can also work with complex vectors. Understand vectors, and math in general, you have to be able to visualize the concepts, so rather than developing the geometric interpretation as an after thought, we start with it. 1.1 vector addition and multiplication by a scalar we begin with vectors in 2d and 3d euclidean spaces, e2 and e3 say. e3 corresponds to our. Linear independence: a set of vectors {v1, v2, –, vm} is said to be linearly independent if none of the vectors in it can be written as a linear combination of the others.
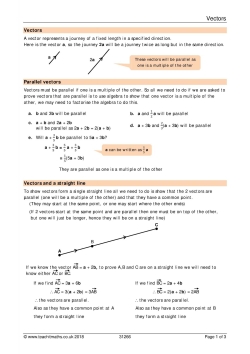
Vectors Review Sheet Higher Gcse Maths Teachit In basic engineering courses, the term vector is used often to imply a physical vector that has “magnitude and direction and satisfies the parallelogram law of addition.” in mathematics, vectors are more abstract objects than physical vectors. like physical vectors, tensors must satisfy the rules of tensor addition and scalar multiplication. Review of vectors we will work with real vectors, meaning vectors for which each component is a real number. with minimal changes, one can also work with complex vectors. Understand vectors, and math in general, you have to be able to visualize the concepts, so rather than developing the geometric interpretation as an after thought, we start with it. 1.1 vector addition and multiplication by a scalar we begin with vectors in 2d and 3d euclidean spaces, e2 and e3 say. e3 corresponds to our. Linear independence: a set of vectors {v1, v2, –, vm} is said to be linearly independent if none of the vectors in it can be written as a linear combination of the others.

Vectors Case Study On Behance Understand vectors, and math in general, you have to be able to visualize the concepts, so rather than developing the geometric interpretation as an after thought, we start with it. 1.1 vector addition and multiplication by a scalar we begin with vectors in 2d and 3d euclidean spaces, e2 and e3 say. e3 corresponds to our. Linear independence: a set of vectors {v1, v2, –, vm} is said to be linearly independent if none of the vectors in it can be written as a linear combination of the others.

Comments are closed.